| Physics of the didgeridoo |
|
On this page we will explore some of the physics of the didgeridoo and answer these questions:
This page is written using some material kindly supplied by Wolfgang Droescher (click here for Wolfgang's original article). First of all lets be clear no amount of physics will be able to describe a termite hollowed didgeridoo. Termite hollowed didgeridoos have a very irregular inner surface unique to each didgeridoo which do give many a genuine didgeridoo very interesting sound characteristics, but make it far too complicated to describe physically. So for the purpose of this page we need to reduce a didgeridoo to a perfect pipe. Consequently this page is really about the physics of a PVC didgeridoo or any other didgeridoo as long as the inside diameter is exactly the same for the whole length of the didgeridoo. Next lets have a look at some basic music theory:
Now that we have covered a bit of basic music theory lets see what actually influences f- the frequency a given pipe resonates at:
Note: The diameter of the pipe is not important at all for this basic calculation; a bigger diameter only makes the pipe sound louder as it gives a higher amplitude. Which leads us finally to the physics formula determining the frequency a certain didgeridoo resonates at (as long as it is a perfect pipe):
Using this formula we have prepared this table that gives you both the frequency of a particular key as well as the length of a PVC didgeridoo in that key.
We need (apart from the hardware) the formula from above, the frequency table on left - and a calculator. l = c / 4f = 344 / (4 x 65.40) = 344 / 261.63 = 1.315 m That's it! The other way round: Let's assume you found a wonderful hollowed branch (which is a perfect pipe) of roughly one meter in length out in your backyard and you are wondering which musical key this "didge" would make. After smoothing the edges a bit you end up with exactly 1.05 m. Take out your calculator and start off: f = c / 4l = 344 / (4 x 1.05) = 344 / 4.2 = 81.90 Hz This is pretty close to E (82.41Hz), so now you have a rough idea which key you can make out of it. Use the formula above to get the exact length for E (1.04 m) then cut off some more millimetres - done! Or simply leave your new didge as is, it's close enough anyway. As we stated earlier our calculations are based on the speed of sound in dry air at 20° C. So what happens if it gets hotter or colder, or wetter? We will ignore humidity here as there is no easy formula to account for it and the speed of sound in air depends mostly on temperature; it can be calculated with this small formula: c = 331.6 + 0.6 x t Examples: This means that the frequency of a 1.32cm long didgeridoo will be about 3.4% higher if the temperature is 40° C, which is halfway between C and C#. As you can see extreme temperature changes will change the key of your didj, but a few degrees warmer or colder than 20° C do not matter much. Wolfgang also reminded me that we are talking about the temperature of the air going through the didj which is your breath. And that does not change that much. Keep on didjing... Read on: |
|
Home | About Us | Checkout | Community | Help | Information | Shopping Didj Forum | Send a Didj Greeting Card | Guest Book |
| © Didjshop.com, 1993-. For rights reserved and granted see our Copyright notice. |

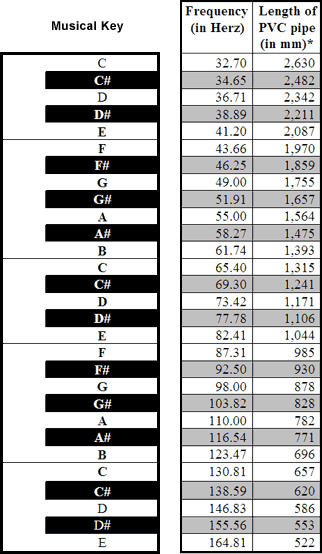 Let's say you want to build a PVC
didge with a musical key of C (as described on
Let's say you want to build a PVC
didge with a musical key of C (as described on